Toric Geometry in Reaction Networks

Azimuth 2018-08-02

I want to figure out how to use toric geometry in chemistry. This is a good intro to toric geometry:

• William Fulton, Introduction to Toric Varieties, Princeton U. Press, 1993.

and this is a great explanation of how it shows up in chemistry:

• Mercedes Perez Millan, Alicia Dickenstein, Anne Shiu and Carsten Conradi, Chemical reaction systems with toric steady states, Bulletin of Mathematical Biology 74 (2012), 1027–1065.

You don’t need to read Fulton’s book to understand this paper! But you don’t need to read either to understand what I’m about to say. It’s very simple.

Suppose we have a bunch of chemical reactions. For example, just one:

$\mathrm{A} \mathrel{\substack{\alpha_{\rightarrow} \\\longleftrightarrow\\ \alpha_{\leftarrow}}} \mathrm{B} + \mathrm{C}$

or more precisely two: the forward reaction

$\mathrm{A} \to \mathrm{B} + \mathrm{C}$

with its rate constant $\alpha_\to,$ and the reverse reaction

$\mathrm{B} + \mathrm{C} \to \mathrm{A}$

with its rate rate constant $\alpha_{\rightarrow}.$ Then as I recently explained, these reactions are in a detailed balanced equilibrium when

$\alpha_{\to} [\mathrm{A}] = \alpha_{\rightarrow} [\mathrm{B}] [\mathrm{C}]$

This says the forward reaction is happening at the same rate as the reverse reaction.

Note: we have three variables, the concentrations $[\mathrm{A}], [\mathrm{B}]$ and $[\mathrm{C}],$ and they obey a polynomial equation. But it’s a special kind of polynomial equation! It just says that one monomial—a product of variables, times a constant—equals another monomial. That’s the kind of equation that’s allowed in toric geometry.

Let’s look at another example:

$\mathrm{B} + \mathrm{C} \mathrel{\substack{\beta_{\rightarrow} \\\longleftrightarrow\\ \beta_{\leftarrow}}} \mathrm{D} + \mathrm{D} + \mathrm{A}$

Now we have a detailed balance equilibrium when

$\beta_{\to} [\mathrm{B}] [\mathrm{C}] = \beta_{\leftarrow} [\mathrm{D}]^2 [\mathrm{A}]$

Again, one monomial equals another monomial.

Now let’s look at a bigger reaction network, formed by combining the two so far:

$\mathrm{A} \mathrel{\substack{\alpha_{\rightarrow} \\\longleftrightarrow\\ \alpha_{\leftarrow}}} \mathrm{B} + \mathrm{C} \mathrel{\substack{\beta_{\rightarrow} \\\longleftrightarrow\\ \beta_{\leftarrow}}} \mathrm{D} + \mathrm{D} + \mathrm{A}$

Detailed balance is a very strong condition: it says that each reaction is occurring at the same rate as its reverse. So, it happens when

$\alpha_{\to} [\mathrm{A}] = \alpha_{\rightarrow} [\mathrm{B}] [\mathrm{C}]$

and

$\beta_{\to} [\mathrm{B}] [\mathrm{C}] = \beta_{\leftarrow} [\mathrm{D}]^2 [\mathrm{A}]$

So, we can have more than one equation, but all of them simply equate two monomials. That’s how it always works in a detailed balanced equilibrium.

Definition. An affine toric variety is a subset of $\mathbb{R}^n$ defined by a system of equations, each of which equates two monomials in the coordinates $x_1, \dots, x_n.$

So, if we ignore the restriction that our variables should be ≥ 0, the space of detailed balanced equilibria for a reaction network where every reaction is reversible is an affine toric variety. And the point is, there’s a lot one can say about such spaces!

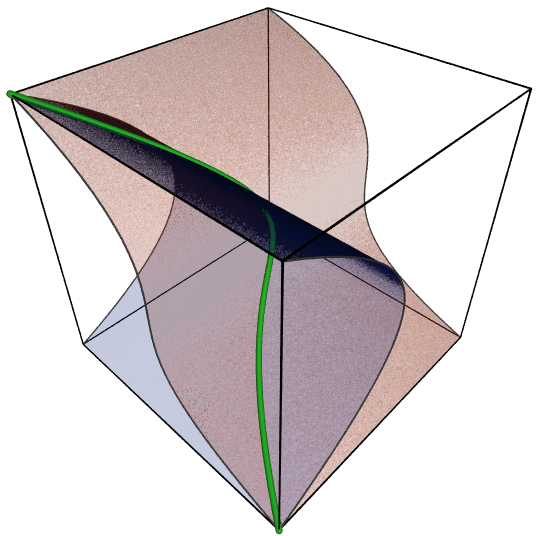
A simple example of an affine toric variety is the twisted cubic, which is the subset

$\{ (x,x^2,x^3) \} \subset \mathbb{R}^3$

Here it is, as drawn by Claudio Rocchini:

I may say more about this, but today I just wanted to get the ball rolling.

Puzzle. What’s a reaction network whose detailed balanced equilibrium equations give the twisted cubic?