Error in Ramanujan’s approximation for ellipse perimeter

Ramanujan discovered an incredibly accurate approximation for the perimeter of an ellipse. This post will illustrate how accurate the approximation is and push its limits.

As with all computations involving ellipses, the accuracy of Ramanujan’s approximation increases as eccentricity increases. But the error increases slowly, and in fact is bounded.

Let a and b be the semi-major and semi-minor axes of an ellipse. Then Ramanujan’s approximation for the perimeter of the ellipse is

where λ = (a − b)/(a + b).

Example

To illustrate how accurate the approximation is, let’s apply it to a very large, highly eccentric ellipse: the orbit of Sedna, a dwarf planet discovered in 2003. Sedna has the most elliptical orbit of any of the dwarf planets, 0.8496 compared to 0.2488 for Pluto. Sedna is also about 12 times further from the sun than Pluto.

The semi-major axis of Sedna’s orbit is 76 billion kilometers. Eccentricity e corresponds to aspect ratio √(1 − e²) (see this post), and so the semi-minor axis is about 40 billion kilometers. Let’s assume the orbit of Sedna is perfectly elliptical, which it is not, and that the semi-axes stated above are exact, which they are not. Then the length of Sedna’s orbit is on the order of 366 billion kilometers, and Ramanujan’s approximation has an error of about 53 kilometers.

Error

Here’s a plot of the relative error when b = 1 and a varies.

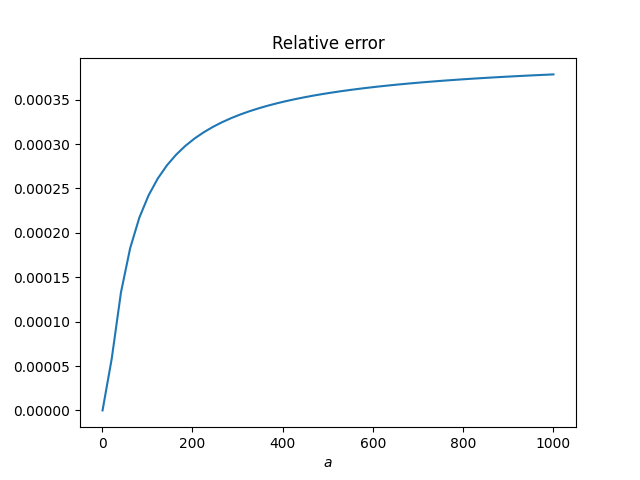
The error appears to be approaching an asymptote, and in fact it is. The error is bound by 4/π − 14/11 = 0.00051227…., as proved here.

The post Error in Ramanujan’s approximation for ellipse perimeter first appeared on John D. Cook.